Problem Study (algebra)

ဒီေန႔ group မွာ ဆရာ ကိုၿဖိဳး ဆရာ ေက်ာ္ေဇျမင့္ တို႔ရဲ့ မွတ္သားဖြယ္ရာ ပုစာၦေတြကို အေျဖႏွင့္တကြ တင္ေပးလိုက္ပါတယ္။ ကၽြန္ေတာ္စဥ္စားမိသလို တြက္ေပးထားတာ ျဖစ္တဲ့အတြက္ ဒီထက္ပိုမို အဆင္ေျပ ေကာင္းမြန္တဲ့ တြက္နည္းမ်ား ရွိမွာ အမွန္ပါ။ ေက်ာင္းသားမ်ားအတြက္၊
ေလ့လာသူအတြက္ တစ္စံုတရာ
အက်ိဳးရွိေစမယ္လို႔ ယံုၾကည္လ်က္....။

Logarithms

 John Napier (inventor of logarithms)

Logarithm ကို အေျခခံက်က်ေလး ရွင္းျပေပးထားလို႔ နားလည္လြယ္မွာပါ။ ေလ့က်င့္ဖို႔ exercises ေတြလည္းပါေတာ့ အသံုး၀င္မယ္လို႔ ေမွ်ာ္လင့္ပါတယ္။

Coordinate Geometry

Problem Study (Trigonometry)

2016 တကၠသိုလ္၀င္တန္း (တိုင္းႏွင့္ျပည္နယ္) သခၤ်ာအေျဖ

Problem Study (Binomial Theorem)

1.   Given that (p - ${\displaystyle \frac{1}{2}}$ x)⁶ = r - 96x + sx² + ... , find p, r, s.

     Solution

      (p - ${\displaystyle \frac{1}{2}}$ x)⁶ = r - 96x + sx² + ...

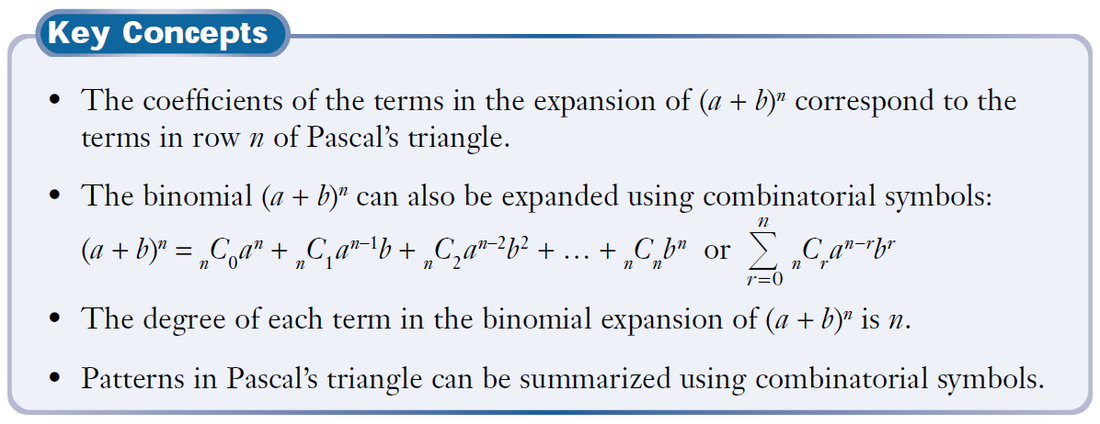
      Using binomial expansion,

      ⁶C₀ p⁶ + ⁶C₁ p⁵ (- ${\displaystyle \frac{1}{2}}$ x) + ⁶C₂ p⁴ (- ${\displaystyle \frac{1}{2}}$x)² + ... = r - 96x + sx² + ...

     
        p⁶ + 6 p⁵ (- ${\displaystyle \frac{1}{2}}$ x) + 15 p⁴ (- ${\displaystyle \frac{1}{2}}$x)² + ... = r - 96x + sx² + ...

      p⁶ - 3 p⁵ x + ${\displaystyle \frac{15}{4}}$ p⁴ x² + ... = r - 96x + sx² + ...

      _∴ 3p⁵ = 96

          p = 2

      r = p⁶ = 64

      s = ${\displaystyle \frac{15}{4}}$ p⁴ = ${\displaystyle \frac{15}{4}}$(2)⁴ =${\displaystyle \frac{15}{4}}$(16) = 60.

2.  The first three terms in the expansion of (a + b)ⁿ in  
      ascending powers of b are denoted by p, q and r 
      respectively. Show that  ${\displaystyle \frac{q^{2 }}{pr}=\frac{2n}{n-<!--\; -\; -->1}}$ . Given that  
      p = 4, q = 32 and r = 96, evaluate n.

      Solution

      (a + b)ⁿ  = p + q + r + ...

        ⁿC₀ aⁿ + ⁿC₁ a^n-1 b + ⁿC₂ a^n-2 b² + ... = p + q + r + ...

      aⁿ + n a^n-1 + ${\displaystyle \frac{n(n-<!--\; -\; -->1)}{2}}$ a^n-2 b² + ... = p + q + r + ...

     ∴ p  = aⁿ 

        q  = n a<sup>n-1

           r  = ${\displaystyle \frac{n(n-<!--\; -\; -->1)}{2}{a}^{n-<!--\; -\; -->2}{b}^2}$ 

     ∴ ${\displaystyle \frac{q^2}{pr}=\frac{{(n{a}^{n-<!--\; -\; -->1})}^2}{a^n\frac{n(n-<!--\; -\; -->1)}{2}{a}^{n-<!--\; -\; -->2}{b}^2} }$

${\displaystyle \; =\frac{2n^2{a}^{2n-<!--\; -\; -->2}{b}^2}{n(n-<!--\; -\; -->1)a^{2n-<!--\; -\; -->2}{b}^2} }$

${\displaystyle \; =\frac{2n}{n-<!--\; -\; -->\mathrm{1 }}}$</sup>

         When p = 4, q = 32 and r = 96, 

          ${\displaystyle \frac{2n}{n-<!--\; -\; -->1}=\frac{32\times <!--\; \times \; -->32}{4\times <!--\; \times \; -->96}}$

          ${\displaystyle \frac{2n}{n-<!--\; -\; -->1}=\frac{8}{3}}$

          8n - 8 = 6n

         2n = 8

         n = 4

  3.     Using binomial theorem, find the coefficient of x² 
          in the expansion of (3 + x - 2x²)⁵.

          Solution 

             [3 + (x - 2x²)]⁵

            = 3⁵ + 5 (3⁴) (x - 2x²) + 10 (3³) (x - 2x²)² + ... 

         = 3⁵ + 405(x - 2x²) + 270 (x² - 4x³ + 4x⁴) + ... 

        ∴ The coefficient of x² in the expansion of (3 + x - 2x²)⁵  

            = 405 (-2) + 270 

            = - 540

4.     Find the coefficient of x⁴ in the expansion of 
        (x² - 5x + 12)${\displaystyle (x-<!--\; -\; -->\frac{2}{x}{)}^6}$.

        Solution 

          (x² - 5x + 12)${\displaystyle (x-<!--\; -\; -->\frac{2}{x}{)}^6}$ 

       = (x² - 5x + 12) (${\displaystyle x^6-<!--\; -\; -->6x^5(\frac{2}{x})+15x^4(\frac{4}{x^2})+...}$) 

       = (x² - 5x + 12) (x⁶ - 12x⁴  + 60x² + . . . )

      ∴ The coefficient of x⁴ = 1(60) + 12 (-12) = - 84 

5.    In the expansion of (1 + x)(a - bx)¹², the coefficient of

       x⁸ is zero. Find the value of the ratio a : b.

       Solution

         (1 + x)(a - bx)¹² 

      = (1 + x)(- bx + a)¹²
 

      = (1 + x) (¹²C₀ (-bx)¹² + ¹²C₁  (-bx)¹¹a +¹²C₂ (-bx)¹⁰a² + ¹²C₃ (-bx)¹⁰a³

                           + ¹²C₄ (-bx)⁹a⁴ + ¹²C₅ (-bx)⁸a⁵+ ¹²C₆ (-bx)⁷a⁶ + ...) 
  
     ∴ The coefficient of x⁸ = ¹²C₅ b⁸a⁵ -  ¹²C₆ b⁷a⁶

     By the problem,

     ¹²C₅ b⁸a⁵ - ¹²C₆ b⁷a⁶ = 0 

    ∴ ¹²C₅ b⁸a⁵ = ¹²C₆ b⁷a<sup>6

     ∴ 12C₅ b = 12C₆ a</sup>  
      
    ∴ ${\displaystyle \frac{a}{b}=\frac{{}^{12}{C}_5}{{}^{12}{C}_6} }$
${\displaystyle \; =\frac{{}^{12}{C}_5}{{}^{12}{C}_5\frac{7}{6}} }$
${\displaystyle \; =\frac{6}{7}}$
Problems Supported by : Sayar Tun Tun Aung